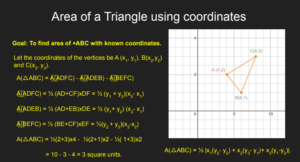
you have been given three points, and you know they are not collinear points and therefore, they will form a triangle. And the question can be how to find the area of triangle using the coordinate system. So, let us try to see that using the coordinate system. So, there is some triangle ABC and I want to find the area of triangle ABC. Let the coordinates of that triangle be ( x1 , y 1 ),( x2 , y2 ) and ( x3 , y 3 ).
Once I have these coordinates, I can plot it here. You can see on the right there is an image of a triangle. Now, how to find the area of this triangle? Now, whatever I discussed so far everything actually relied on dropping a perpendicular to X – axis and finding the area of the geometric object that is formed. In earlier cases, it was just a triangle. Now, if we follow that theory then you can easily see that I need to do something like dropping a perpendicular to x axis. So, I have dropped perpendiculars to X – axis.
Now, I have generated some figures. What are the figures that I have generated? In particular, I have generated 3 trapeziums, trapezium ADFC that is the biggest one encompassing everything. Then, you can look at trapezium ADEB, then you can look at the trapezium BEFC.
Now, my triangle is trapped in between these trapeziums. So, let us try to make our understanding crystal clear. If I want to find the area of triangle ABC, then I need to first consider the biggest possible quadrilateral or trapezium that is ADFC and eliminate the areas of two smaller trapeziums that is ADEB and BEFC. And whatever I am left with is the area of triangle ABC.
Now, do I know how to find the area of trapezium? Yes, I know. The formula is half times sum of parallel sides into the height of the trapezium. So, we need to quantify how will these quantities be calculated? Let us consider trapezium ADFC, if I consider a trapezium the ADFC then what are the parallel sides of this trapezium? Side AD and side FC. So, I will take average of these two parallel sides that is half of AD plus FC. Then, what is a height? Height should have a perpendicular distance, so that is X – axis. So, I know the distance will be DF.
So, let us take the general coordinate system rather than using this coordinate system. What are the coordinates of A and D? So, A has coordinates ( x1 , y 1)and after dropping a perpendicular on X – axis the y coordinate will vanish and therefore, the coordinate of D will be ( x1 ,0). So, what will be the length of AD? It will be purely in terms of y that is y 1 . Similar, thing is applicable for CF. So, it will be nothing but y 3 ; so, area of ADFC,
Area( ADFC)=1 /2 ( AD+FC) ×DF= 1 /2 ( y1+ y 3)× DF.
Now, what is the length of line segment DF or FD? Highest minus the lowest. So, in this case our F is (8 ,0) or ( x3 ,0)and the point D is ( x1 ,0). So, it is ( x3−x1 ).
Area( ADFC)= 1 /2 ( AD+FC)×DF= 1 /2 ( y1+ y 3)×(x 3−x1 )
In a similar manner, I can actually see a smaller trapezium that is ADEB, smaller quadrilateral that is ADEB and the height of that quadrilateral will be the length of ED which is 2 in this case or x2−x1 in the coordinate system. So, this is what our understanding of the length is. In a similar manner, the sum of lengths of parallel sides is y 1+ y 2 .
Area( AD EB)= 1 /2 ( AD+EB)× D E= 1 /2 ( y 1+ y2 )×( x2−x1 ) .
In a similar manner you can compute BEFC.
Area( BEFC)= 1 /2 (BE+CF)×E F= 1 /2 ( y2+ y3 )×( x3−x2
Now, using this you can compute the area of the triangle which can be easily seen to be in this form. So, I have just taken this example and computed these values. So, the values are effectively in this particular case the length of AD was 2.
The length of CF was 3 so
Area (abc )= 1 /2 (2+3)×4− 1 /2 (2+1)×2− 1 /2 (1+3)×2=10−3−4=3 square units.
Now, if you look at this particular thing and rewrite this expression you will get a very nice expression. You can juggle with this expression and try to simplify it by taking a cross products and you will come up with the expression of this form.
A( ∆ ABC)= 1 /2 ∨x1 ( y2−y3 )+x2 ( y3−y 1)+x 3 ( y1−y 2 )∨¿
The absolute sign is just to ensure that the area value should not be negative, but the calculation still remains same. And you need to consider one caution here that all the vertices of a triangle in an anticlockwise direction then only this formula is valid. So, I have considered area of a triangle.
Now what we have seen so far is given two points how to find the distance between two points, given three points if they are collinear, we have found the section formula that can help us to find their ratios or the coordinates of the middle point. Now, if the points are non- collinear, we have seen how to compute the area of the triangle using the coordinate system.