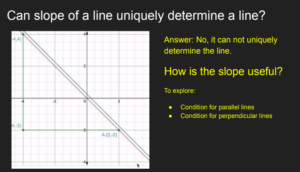
Now the question can be asked that if a line is given to me, I can uniquely determine the slope, but if a slope is given to me can I uniquely determine a line? That is the next question that I will put up. In any sense the question asks can there be many lines with same slope?
If you look at this image closely what we have done is? We have fixed one line and we know how to compute the slope of this line we have a it will be minus 1 based on the coordinates. Now, the blue line that is revolving around is actually having the same inclination as the orange line.
Now, the orange line and blue line have the same inclination; that means, tan of those inclinations will be same, will match and hence there can be infinitely many parallel lines which have a same slope. So, the answer to this question, can slope of a line uniquely determine a line? The answer is no, you cannot uniquely determine a line given the slope of a line or the inclination of the line.
Now, why do we study the concept of slope or whatever we studied how it is helpful? The helpfulness of this concept is just what we discussed in this graphical image, what we are seeing is if the inclinations are same the line better be parallel. So, for parallel lines I can use this concept and derive a condition of slope. Similarly, I can do by rotating them by 90 degrees; that means, I we can consider the perpendicular lines and I can consider general two lines intersecting each other and see what condition I can derive based on the slope.
So, I want to explore the usefulness of slope. So, to explore this I will first figure out the condition for parallel lines and I will figure out the condition for perpendicular lines, in due course we will find the relation between slopes of two lines and their intersection and their angles of intersection. This is what we will do in next few minutes.

So, let us go to the next characterization of parallel lines via slope. Now as you can see in this image there are two parallel lines, they have same inclination, but they are not unique that is what we figured out. So, if I play this video you can see again, this is similar to what we have seen in the last video.
So, I have something which is moving around and there can be infinitely many lines, what remains constant is the inclination, the inclination is same if I have parallel lines. So, let us try to see whether we you can derive something. So, let to put it in a proper context. Let orange line be l 1and the blue line be l 2 be two non-vertical lines. Why non-vertical lines?
Vertical lines have angle of 90 degrees for which the concept of slope is undefined, inclination 90 degrees for which the concept of slope is undefined. So, what I need is non- vertical lines. So, considered two non-vertical lines with slopes m1∧m2given the slopes their inclinations αand βrespectively.
Now, if you have been given that l 1 is parallel to l 2 then α=β, inclinations are same that is what we have seen in the figure and that is what we discussed in the last slide also. So, if α=β then naturally tanα=tan β, once tanα=tan β; what is tanα? It is the slope of line11 that is m1 and tan βis the slope of line l 2which is m2 . Therefore, clearly the slopes are equal, m1=m2 .
The converse that is assumed that, if the slopes are equal then tanα=tan βby a definition. Now, tanα=tan βdoes that imply α is equal to β? In our case because we are restricting the inclinations to vary from 0 to 180 degrees the value of tan is uniquely determined. And therefore, because α∧βlie in 0 to 180 degrees α=β which resolves the problem; that means, their inclinations are same. That means the two lines are parallel. So, l 1 is parallel to l 2 .
So, what is a characterization of parallel lines? That means, if I want to say two non-vertical lines l 1 and l 2 are parallel then it suffices to check whether their slopes are equal or not. If they are parallel then the slopes better be equal and if the slopes are equal then we have parallel lines. Now similar characterization we are searching for in perpendicular lines. So, let us go and try to figure out this characterization for perpendicular lines.
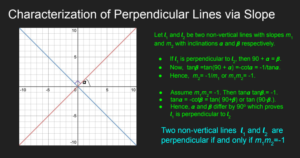
Let us try to visualize, what are the perpendicular lines? So, here are two perpendicular lines one l 1and l 2 let us take the orange line as l 1 and blue line as l 2 . So, l 1 will have slope m1 , l 2 will have slope m2 angle of inclination of l 1
is α then inclination of β, if it is perpendicular to line l 1 is 90+α which is β. And, then you may play with the tangent function of it and you can get something which is very interesting.
So, let us try to figure out what is that interesting thing that we are getting. So, to put it formally let l 1 and l 2 be two non-vertical lines because I cannot work with vertical lines θ equal to 90 degrees, the concept of slope is not defined which slopes m1 , m2 inclinations α and β respectively, no problem in this. If l 1 is perpendicular to l 2 as is the case in this a figure
I have β is equal to90+α. So, if I want to figure out the relation between the slopes of 1 1 and l 2 then it is a good idea to take tangent of β. So, let us take that. So, tan β=tan(90+α) , but tan(90+α) if you use that simple formula that is available to you is −cot α which also can be written as −1 / tan α
But what is tanα? tan β is the slope of a line l 2 which is m2 and tanαis the slope of a line l 1 which is m1 . So, what we have just now derived is m2= −1/ m1 or m1m2=−1. That means, if you take two slopes if you take slopes of two lines take a product of them and if you get the quantity to be equal to −1; that means, you have got a perpendicular line.
But right now, we have not proved that result, what we have proved just now is if l 1 is perpendicular to l 2 then the product of the slopes better be -1. Now I want to prove if the product of the slopes is -1 then the lines are perpendicular, how will I go about this? Exactly the way we went for parallel lines.
So, m1m2 is−1 then I; obviously, tanα tan β=−1; that means, tan βwill be equal to −1 /tan α or tanα=−cot βbut what is −cot β? tan(90+β )or either it will be this way or it will be the other way so, tan(90−β). So, −cot β is either tan(90+β ) or tan(90−β), in any case the difference between α and β is 90 degrees.
Therefore, l 1 is perpendicular to l 2 . Hence, we have proved a characterization that if two non- vertical lines are perpendicular to each other, the product of their slopes is equal to -1 which can be written in this form. Two non-vertical lines l 1 and l 2 are perpendicular if and only if m1m2=−1 or you can verbally write product of their slopes is equal to – 1.
So, this is the characterization of the perpendicular lines via slope. So, what we have seen so far is the characterization of parallel lines by slope and characterization of perpendicular lines via slope, what if they are not parallel or perpendicular and they intersect just like that? If they are not parallel then they better intersect each other.
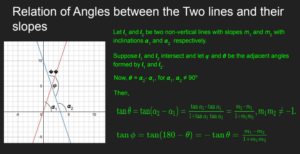
So, in general if I want to have an intersection of two lines and I know the slopes of those two lines. Can I talk about the angle of intersection of these two lines? The answer is yes. So, here is the relation of angles between the two lines and their slopes. So, what I want to say if once I show the figure it will be clear.
As of now let us understand I have two non-vertical lines with slopes m1 and m2 , inclinations α1 and α2 respectively. And, l 1 and l 2 intersect each other, they are not parallel so they will intersect somehow and they are not perpendicular also. So, they intersect in angles φ and θ are the adjacent angles that are formed by l 1 and l 2 , if they intersect in a perpendicular manner the adjacent angles will be 90 degrees each. So, that is not an interesting case because we have resolved that case.
So, now, if they intersect at any angle then this figure will look like this; let us first understand this figure. So, there are two lines l 1 and l 2 . So, l 1 has angle of inclinationα1 , l 2 has inclination α2 these two lines intersect over here near y coordinate φand they have two angles; one is θ, another one is φ. So, these two angles are adjacent angles. What can you say about the angle θ that is formed?
As you can see the angle α2 is obtuse and α1 is slight acute. So, the angle θ is actually α2 minus α1 provided α1 and α2are not equal to 90 degrees. Why? Because I cannot consider vertical lines as simple as that. So, the angle is 90 not equal to 90 degrees, θ=α2−α1 .
So, if I want to talk in terms of slopes of these lines, I better take tangent function and apply it to the angle θ. So, let me do it. So tanθ=tan(α2−α1 ). Take a standard trigonometric formula of tan(α2−α1 ), you will get tan α2−tan α1 /1+tanα1 tan α2 . But what is tanα2? tanα2 is nothing but the slope of line l 2 which is m2 and tanα1 it is slope of line l1 which is m1.
Therefore, the answer to this is m2−m1 /1+m1m2 . So, I know what is tan θ, now you can look at the angle φ which is 180−θ. So, I can similarly derive a relationship for tanφwhich is tan(180−θ), we have already seen, this is −tanθ .So, that m2−m1 will be swapped to m1−m2 denominator remains the same, the condition m1m2≠−1remains the same because they should not be perpendicular.
In this case we have figured out what is the relation of tan of that angle with respect to the slopes of the lines. So, this finishes our discussion on two lines. Now another interesting question that comes is, what if the three points are collinear, then how will the slopes be interpreted? Imagine three points are collinear then what happens is their slopes must be equal because they are all lying on the same line right and there is one common point.
So, if A, B, C are collinear slope of AB is equal to slope of BC and therefore, all of them must be collinear. So, if there is any common point in between those three points the slopes are equal, the points are collinear, that is called the relation of collinearity using slopes.